Computational Fairy Tales

Posted on 2012-03-17 by M. Eric Carr

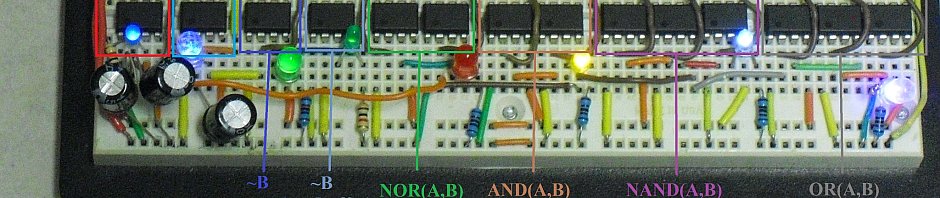
I love finding innovative ways to teach important STEM concepts. As much as I love electronics and everything you can do with it, even I understand that learning about Norton-Thévenin equivalents and pole plots can sometimes be quite dry. As much fun as programming can be, learning about loop invariants, order-of-operations, and the various types of data structures can be rather dull if not presented well.

Computational Fairy Tales, by Jeremy Kubica, is a really cool concept (teach the fundamentals of computer science by making the relevant concepts into fairy-tale plot devices) — and equally importantly, well-executed. Abstract concepts like functions, constants, and linked lists become much more memorable when told in the context of an adventure story or anecdote. Some of the stories, like the time the guards at the north gate learned the difference between assignment and equivalence ( = vs ==), are a hoot.

Posted in Coding, Humor, Resources | Leave a comment

Flying sphere

Posted on 2012-03-16 by M. Eric Carr

Every so often, you come across something awesome but seemingly obvious in retrospect. The Flying Sphere, developed by Japan’s Ministry of Defense, definitely qualifies. It has a single propeller which provides both lift and propulsion. Attitude control and navigation is handled by a series of fins.

It’s more easily understood when seen in action than by reading a description, though…

I wish I’d thought of it — but there’s no reason why I can’t build one!

Posted in Aviation, Current Events, Robotics | Leave a comment

Dirac Delta: Science and Engineering Encyclopedia

Posted on 2012-03-15 by M. Eric Carr

Wikipedia is a good source for general knowledge — but sometimes, more specialized information is needed. Dirac Delta is an interesting site, full of definitions and explanations of many scientific and engineering-related topics. (…and yes, they do have an entry on the Dirac Delta function.)

 

 

Posted in Resources | Leave a comment

Cracking the Capacitor Code

Posted on 2012-03-14 by M. Eric Carr

Small capacitors can sometimes be difficult to read. On large electrolytic capacitors, manufacturers often provide lots of specs, including not only a clear value (10uF, 220uF, etc), but rated voltage and sometimes other information, as well.

On smaller capacitors, this information is often quite terse. Larger ceramic and tantalum capacitors may still have the value written out in full (1uF etc) — but on smaller capacitors, space is at a premium and manufacturers resort to numeric shorthand to show values.

Typically, this shorthand will be one of several kinds:

  • Straight numeric value, with the unit assumed to be pF (picofarads);
  • Numeric value in pF with an “R” standing for a decimal (“2R2”);
  • Exponential format (described below).

The first two types are fairly straightforward — although the lack of standardization can lead to confusion.

Various types of capacitors, most with cryptic markings. (Click for larger.)

As examples, the values of the capacitors in the above picture can be interpreted as follows (top to bottom, left to right:

  • 1000uF electrolytic (= 1mF; value shown directly)
  • 0.47uF electrolytic (value shown directly)
  • 15uF tantalum (15 x 10^6 pF = 15,000 nF)
  • 0.01uF tantalum (10 x 10^3 pF = 10 nF)
  • 22pF ceramic (value in pF marked directly)
  • 150pF ceramic (15 x 10^1 pF)
  • 820pF ceramic (82 x 10^1 pF)
  • 0.16uF ceramic (16 x 10^4 pF, or 160nF)

…of course, the most reliable way to tell for certain is to use a capacitance meter.

 

Posted in Analog, Components, Electronics, HOW-TO | Leave a comment